前段時間閑暇的時候看到一個貝塞爾曲線算法的文章，試著在小程序里去實現小程序的貝塞爾曲線算法，及其效果。

主要應用到的技術點：
1、小程序wxss布局，以及數據綁定
2、js二次bezier曲線算法

核心算法，寫在app.js里

bezier: function (points, times) {

 // 0、以3個控制點為例，點A,B,C,AB上設置點D,BC上設置點E,DE連線上設置點F,則最終的貝塞爾曲線是點F的坐標軌跡。

 // 1、計算相鄰控制點間距。

 // 2、根據完成時間,計算每次執行時D在AB方向上移動的距離，E在BC方向上移動的距離。

 // 3、時間每遞增100ms，則D,E在指定方向上發生位移, F在DE上的位移則可通過AD/AB = DF/DE得出。

 // 4、根據DE的正余弦值和DE的值計算出F的坐標。

 // 鄰控制AB點間距

 var bezier_points = [];

 var points_D = [];

 var points_E = [];

 const DIST_AB = Math.sqrt(Math.pow(points[1]['x'] - points[0]['x'], 2) + Math.pow(points[1]['y'] - points[0]['y'], 2));

 // 鄰控制BC點間距

 const DIST_BC = Math.sqrt(Math.pow(points[2]['x'] - points[1]['x'], 2) + Math.pow(points[2]['y'] - points[1]['y'], 2));

 // D每次在AB方向上移動的距離

 const EACH_MOVE_AD = DIST_AB / times;

 // E每次在BC方向上移動的距離 

 const EACH_MOVE_BE = DIST_BC / times;

 // 點AB的正切

 const TAN_AB = (points[1]['y'] - points[0]['y']) / (points[1]['x'] - points[0]['x']);

 // 點BC的正切

 const TAN_BC = (points[2]['y'] - points[1]['y']) / (points[2]['x'] - points[1]['x']);

 // 點AB的弧度值

 const RADIUS_AB = Math.atan(TAN_AB);

 // 點BC的弧度值

 const RADIUS_BC = Math.atan(TAN_BC);

 // 每次執行

 for (var i = 1; i <= times; i++) {

 // AD的距離

 var dist_AD = EACH_MOVE_AD * i;

 // BE的距離

 var dist_BE = EACH_MOVE_BE * i;

 // D點的坐標

 var point_D = {};

 point_D['x'] = dist_AD * Math.cos(RADIUS_AB) + points[0]['x'];

 point_D['y'] = dist_AD * Math.sin(RADIUS_AB) + points[0]['y'];

 points_D.push(point_D);

 // E點的坐標

 var point_E = {};

 point_E['x'] = dist_BE * Math.cos(RADIUS_BC) + points[1]['x'];

 point_E['y'] = dist_BE * Math.sin(RADIUS_BC) + points[1]['y'];

 points_E.push(point_E);

 // 此時線段DE的正切值

 var tan_DE = (point_E['y'] - point_D['y']) / (point_E['x'] - point_D['x']);

 // tan_DE的弧度值

 var radius_DE = Math.atan(tan_DE);

 // 地市DE的間距

 var dist_DE = Math.sqrt(Math.pow((point_E['x'] - point_D['x']), 2) + Math.pow((point_E['y'] - point_D['y']), 2));

 // 此時DF的距離

 var dist_DF = (dist_AD / DIST_AB) * dist_DE;

 // 此時DF點的坐標

 var point_F = {};

 point_F['x'] = dist_DF * Math.cos(radius_DE) + point_D['x'];

 point_F['y'] = dist_DF * Math.sin(radius_DE) + point_D['y'];

 bezier_points.push(point_F);

 }

 return {

 'bezier_points': bezier_points

 };

 }

注釋很詳細，算法的原理其實也很簡單。 源碼也發出來吧，github地址:https://github.com/xiongchenf/flybus.git

調用方法和用法就不占篇幅了，都是基礎的東西。效果圖如下：

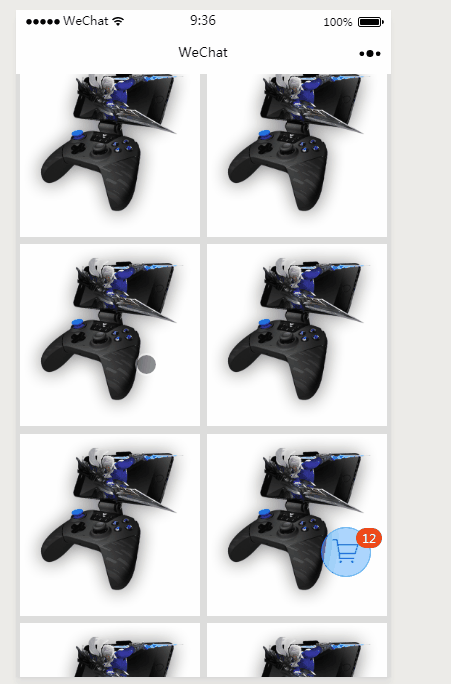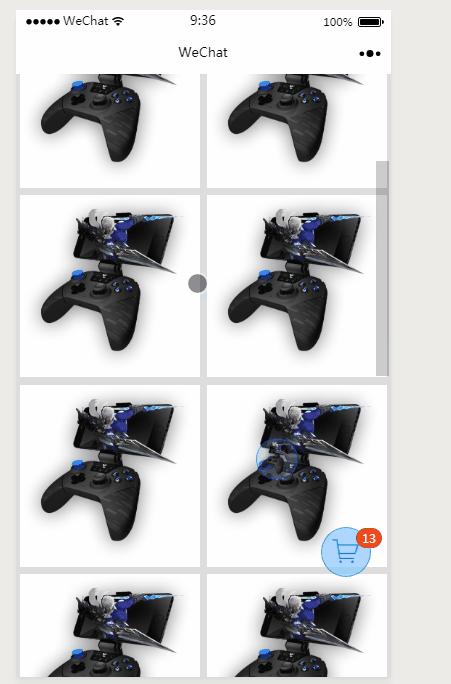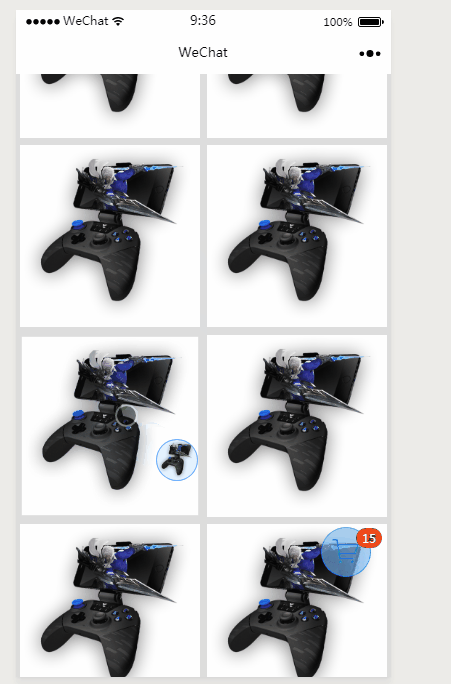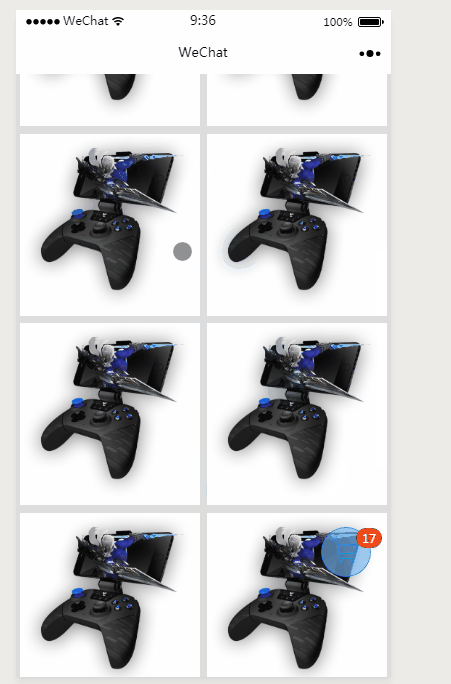
094614lq8csndfksittquq.gif